Sunflower HexChess
I was browsing the web on the subject of "tessellating the Euclidean Plane" when I came across this page on hexagonal tessellations. Hexagons and hexagonal chess variants hold a certain fascination for me and I was particularly drawn to the radial tessellation centred on an octagon. Serendipitously I had also just been reading about Circular Chess, and so was born the idea of creating an hexagonal variant with a circular motif.ÂDue to the increasing number of cells rather than an increasing size of cells, as we move outward on the board, the resulting game might be better termed "radial chess" rather than "circular".
Setup
| The board consists
of 120 hexes, each in one of three colours and each with a border in
one of 5 colours. There are 40
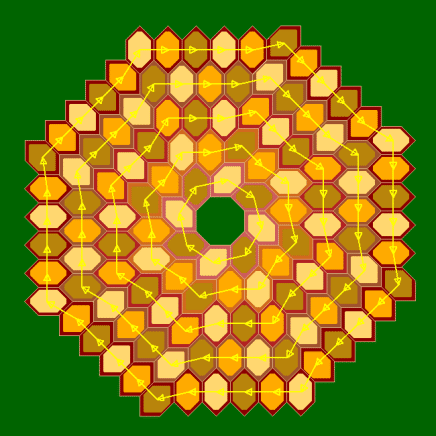
hexagons of each colour. The exact colours used for the board cells are immaterial though the images here use three yellowish hues: a pale yellow; a golden yellow; and a dark greenish yellow. The five border colours help in defining the topology of the board. Again, the precise colours are irrelevant with the images here using shades of red and brown. The board reminds me somewhat of a sunflower , hence the name of the variant. |
|
 |
 |
| The
120 hexes are
arranged in five paths or tracks that encircle the central
octagon and the cells in each path have the same colour border.Â
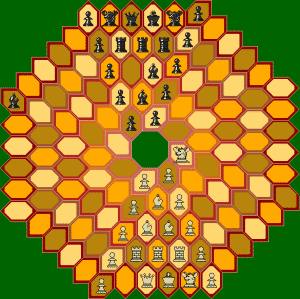
These paths are illustrated by the image above-left. The inner path consists of 8
cells with a light red border.
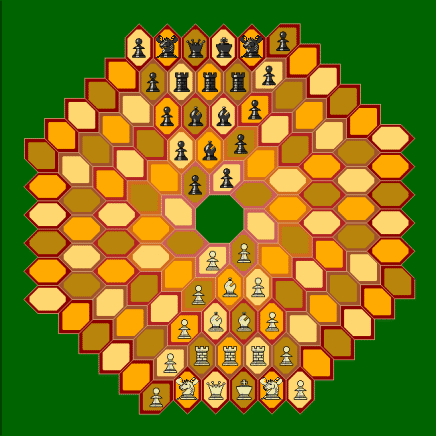
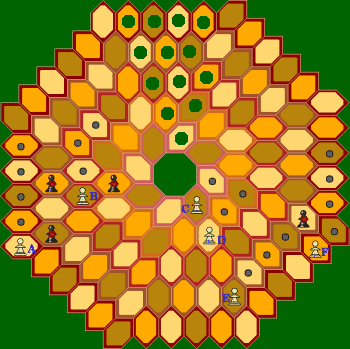
The mid-inner path consists of 16 cells with a light brown border. The middle path consists of 24 cells with a red border. The mid-outer path consists of 32 cells with a dark brown border. The final 40 cells with a dark red border form the outer path. Movement around a single track, clockwise or anti-clockwork, is permitted and is classed as orthogonal movement The image above-right shows the starting position of the pieces. |
|
Notation
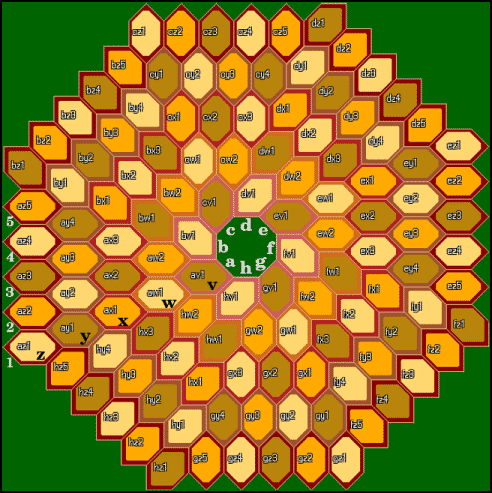
As well as being arranged in the 5 tracks, the
cells of the Sunflower board can be divided into 8 sectors. In
each of these the 15 cells that
make up the sector all have the same alignment.
The
image on the left demonstrates how
this division into sectors is used to
produce the individual cell references.Â
Each sector is referred
to by a letter, a,
b, c, d, e, f, g, or h. Sector (a) consists of the 15
cells aligned East to West and expands outwards to the West or Left,
from White's perspective. The remaining sectors are labelled from
Sector (a) in a clockwise direction.
Each sector has a prime radial of 5 cells, one on each track, running
from the centre to
the outer path.Â
These cells, starting from the centre, are
assigned a letter, v, w, x, y,
and z.
Finally there are up to 5
cells running clockwise along each path from one prime radial to the
next. These "path segment" cells are numbered clockwise from 1 to
5.
Each cell reference thus consists of exactly 3 characters, the sector
letter followed
by the prime radial letter followed by the path segment number.Â
These individual cell references are also shown in the left-hand image.
At the start of a game the white pieces occupy all of sector {g} plus the prime radial of sector {h}. The black pieces occupy all of sector {c} plus the prime radial of sector {d}.
Pieces
Orthogonal and Diagonal Movement
|
|
|
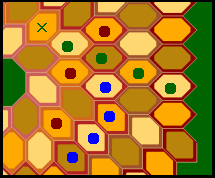
An orthogonal step is taken from the current cell to an adjacent cell joined along a cell edge.
A series of orthogonal steps must conform to the following rules.
- The first step will cross two borders of either the same colour or of differing colours. Subsequent steps must follow the pattern set by the initial step in respect of the borders. Thus if the first step crosses same-colour borders, any subsequent step forming part of the same move must also cross same-colour borders. This will be the case when a piece is moved along one of the five tracks.
- When the series of steps crosses different-colour borders, the exit edge from a hex must be directly opposite the entry edge.
The red dots form a diagonal series while the blue (same-colour borders) and green (different-colour borders) form two orthogonal series. Note that the cell marked by a green cross cannot form part of the green dot series.
Piece Movement
 Pawn - each player starts with 10
|
 Bishop - each player starts with 3
|
 Rook - each player starts with 3
|
 Dragoon - each player starts with 2
|

Combines the moves and captures of Rook and Bishop |
 King- each player starts with 1 Moves 1 hex in any direction. Cannot move into a hex that is attacked by an opponent's piece. |
Rules
The two sides are White and Black, moving alternately with White moving first.Each player starts with 20 pieces:
A player wins if:
- The opponent's King is checkmated.
- The opponent is left with only a King, all other pieces having been captured.
- The opponent is unable to make a legal move (stalemate).
- The opponent's last move causes the third repetition of a
position.
Notes
Although partly inspired by Circular chess this variant is not an attempt to create an hexagonal equivalent of that game. Rather it is an attempt at using a delightful hexagonal tessellation as the basis for a game board. Any attempt at transferring the circular game into an equivalent hexagonal format would need at least to keep the same number of cells in each encircling ring, rather than the increasing number as seen here.This increasing number of cells, with 40 hexes in the outer track, is the main reason for the restriction on orthogonal movement as I wanted to maintain a balance between the strengths of bishop and rook. As the longest diagonal and the longest non-path orthogonal is 5, a maximum move of 5 hexes might seem the obvious choice. However I wanted to keep the rooks slightly stronger than the bishops as is the usual case in chess. On a board with hexagonal cells increasing the obvious 5 to a maximum of 6 seemed appropriate.
The length of the outer tracks also explains the enhanced pawn movement. Without the increase in movement allowed to the pawn on the outer paths progress from starting position to promotion would be just too slow.
I was also unhappy with the knight's move on a board which is effectively quite narrow and which thus prevents a knight from achieving it's maximum move potential. So I devised a leaping piece better adapted to the board's topology to replace the knight, which I have called a Dragoon. Originally dragoons were mounted infantry, able to defend a position or ride to the point of conflict before dismounting to fight on foot. So the Dragoon is able to move, but not capture, by stepping 1 cell in any direction, or move and capture by leaping 2 or 3 cells in any direction. The icon used here for the Dragoon is the orthodox knight backed by a pair of crossed cavalry sabres.
Playing Tips
Fool's Mate
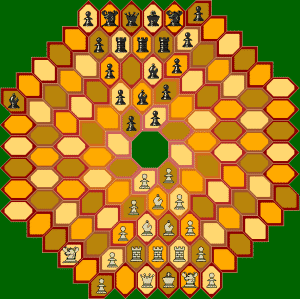
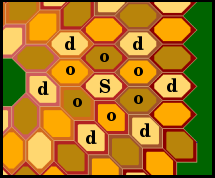
Checkmate by White is possible in just 2 moves, as illustrated by the following images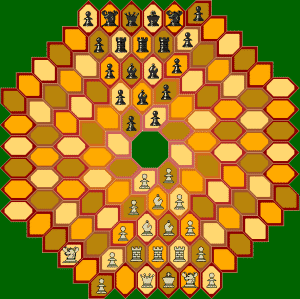 |
 |
 |
| 1. Dragoon
gz5 - hz3 |
1... Bishop cx2 - bz1 | 2. Dragoon hz3 - fv1 # |
Relative Piece Values
|
|
Pawn |
Bishop
|
Rook |
Dragoon
|
Queen |
Suggested value |
1.0 |
3.0 |
4.5 |
6.0 |
7.5 |
 This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
By Graeme C Neatham.
Web page created: 2006-12-22. Web page last updated: 2006-12-22
 Moves by advancing up to a
Moves by advancing up to a 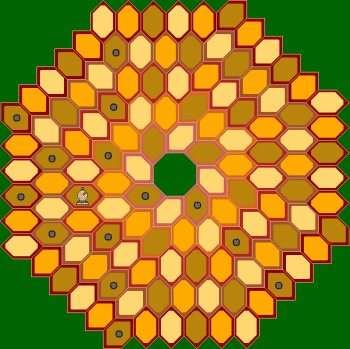 Moves or Captures by sliding any number of hexes
along a diagonal but may not
jump over
other pieces.
Moves or Captures by sliding any number of hexes
along a diagonal but may not
jump over
other pieces.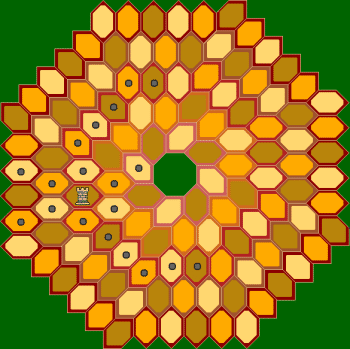 Moves or Captures by sliding up to a maximum of
six hexes along
an orthogonal but may not jump over
other pieces.
Moves or Captures by sliding up to a maximum of
six hexes along
an orthogonal but may not jump over
other pieces.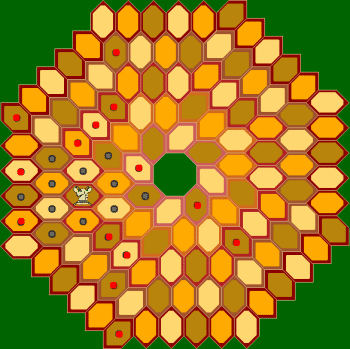 Moves 1 hex in any direction into an
Moves 1 hex in any direction into an 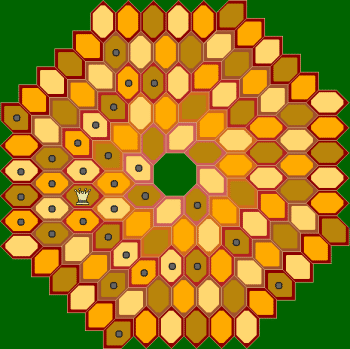 Queen- each player starts with 1
Queen- each player starts with 1