Chad
The game Chad was invented in 1979 by Christian Freeling. According to Pritchard in The Encyclopedia of Chess Variants, Chad was frequently played in The Fanaat, a games club at the Technical University of Twente, Enschede, the Netherlands, and was featured in The Gamer.
The description below is taken from the website of Christian Freeling and Ed van Zon (with permission) (slightly edited by me); and also the pictures were taken from this website, called Mindsports.
See the Mindsports website for more information on Christian Freeling's other chess variants, methods to play several of these on the Internet against others, and more. There also is a variant on a board make of hexagons: HexChad.
Rules
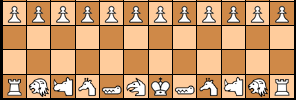
The initial position is shown below. Chad is played on an uncheckered board of twelve by twelve squares, turned 45 degrees. Squares are denoted by their usual coordinates, with a1 denoting the bottom corner, a12 the right corner, l1 the left corner, and l12 the upper corner in the description below.
 White:
White:
King d4; Rook c3, c4, c5, d3, d5, e3, e4, e5.
Black:
King i9; Rook h8, h9, h10, i8, i10, j8, j9, j10.
The areas covered by the pieces are called the castles. Each castle has twelve adjacent squares that together constitute the wall. So, the walls are respectively b3, b4, b5, c2, d2, e2, c6, d6, e6, f3, f4, and f5 for the walls of white, and g8, g9, g10, h7, i7, j7, h11, i11, j11, k8, k9, and k10.
White begins. Players move, and must move, in turn.
The King is confined to his 3x3 castle. He may go and capture using either the King's move or the Knight's move.
It's customary to look at the King in terms of the squares it does not cover. In the center it covers the whole castle, on the side he does not cover the square on the opposite side, and in the corner it does not cover the other corner squares.
The rook moves like the rook in Chess, unhindered by castles and walls. If a rook ends its move inside the opponent's castle, it is promoted to Queen.
The Queen moves like the Queen in Chess, unhindered by castles and walls.
The mutual right of capture exists, and only exists, between an attacking piece on the wall and a defending piece inside the castle. Apart from this situation pieces simply block one another.
This is a crucial rule! It is illustrated in the next diagram.
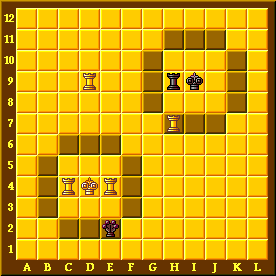
Black's castle shows a rook on the wall facing a defender inside. In such a situation both have the right to capture. However, in this specific situation only white can capture because the black rook is pinned! This position shows one of the basics of attack. If it were white's turn he can mate in two. White's castle shows a black Queen able to capture either rook and one of them able to capture the Queen. If it were black's turn however, he has better than that, because he too can mate in two!
Tactics
A few words on basic tactics: Consider the position around black's castle in the diagram above, disregarding the other pieces and assuming it is part of an actual position. Of course it is black's turn, otherwise white would mate in two. What can black do? Interposing a piece on any of the squares between the black rook and either of the white rooks, would parry the immediate threat. If this isn't possible, black's only option is to move the defending rook towards the pinning one. Needless to say that the white rooks illustrate a basic attacking pattern. It appears in a variety of forms in almost all attacking concepts.
Another basic concept is the promotion sacrifice. It derives from the fact that an attacker, once he is inside the castle (and thus automatically a Queen), can only be captured by the King. A King on the side leaves one square unprotected, and a King in the corner three. The sacrifice of a piece to force the King to the side or into the corner, to clear the way for a second piece to promote on an unprotected square, is very common. A Queen is worth the sacrifice of a piece anytime!
In positional respect, a rook on a square diagonally adjacent to the enemy castle covers two segments of the wall. Needless to say these spots are popular. Finally, every attack eventually draws from defending forces, so a mating attack should drive home. If it fails, 3-fold is one's only hope!
Comments
It is planned to feature Chad in the `Arena' of Mindsports, which means that then it is possible to play this game against others on the Internet using the well-crafted interface that is used on the Mindsports website to play several other games. Pritchard gives a Chad problem `White mates in 7' and comments: `the problem ... reveals something of the game's beauty'. The inventor called the game (in Dutch): `simple but strategic very deep' in an email to me.
Rules written by Christian Freeling for the Mindsports Website, slightly edited for the Chess Variant Pages, and introduction and comments added by Hans Bodlaender. Rules and images copyright Mindsports; used here with permission.
WWW page made: December 16, 1997. Last updated: January 23, 2005.