[ List Earliest Comments Only For Pages | Games | Rated Pages | Rated Games | Subjects of Discussion ]
Single Comment
 Philip & John Ry wrote on Sat, Dec 14, 2002 06:03 AM UTC:
Philip & John Ry wrote on Sat, Dec 14, 2002 06:03 AM UTC: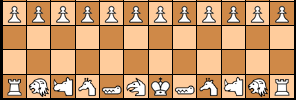
Check out Grant Acedrex, our featured variant for April, 2024.
 Philip & John Ry wrote on Sat, Dec 14, 2002 06:03 AM UTC:
Philip & John Ry wrote on Sat, Dec 14, 2002 06:03 AM UTC: