Piececlopedia: Andernach Grasshopper and Friends
Historical notes
The Andernach Grasshopper and related pieces were invented by the author in 2000.I don't remember exactly when I invented the AG. I had been kicking the idea around in my brain for some time when I emailed Gianni Donati - then the fairies editor of StrateGems chess problem magazine about it on July 26, 2000. Originally I had suggested the idea as a fairy condition, since I'd already noticed that it could be applied to any piece that hurdles other pieces, but we both came to the conclusion that it was really a new piece, and the Andernach-Grasshopper was born.
Three days later I invented the Einstein-Grasshopper and the Circe-Grasshopper, which follow logically from the Andernach-Grasshopper. They are also described below. They have not attracted the attention that the Andernach-Grasshopper has, however.
In October of 2000 the Good Companions Meeting was held in Indiana, and one of the activities there was a quick-composing tourney using the AG. The results of the tourney are discussed in StrateGems issue 13, Jan-Mar 2001. The winner was Milan R. Vukcevich and his problem is shown below.
In the 2001-2002 Composing Match between the USA and Great Britian, one of the sections was to compose a helpmate of any length using the AG. First prize in this section went to David L. Brown of the USA; his problem is also shown below. The results of this tourney should appear in an upcoming StrateGems issue.
StrateGems helpmate editor Dan Meinking has been very helpful in promoting the AG. He was the captain of the USA team in the Composing Match, and he is the author of the first published regular-issue AG problem. It appeared in StrateGems issue 17, Jan-Mar 2002. The problem is shown below and the solution appeared in SG19.
In the fall of 2001, the AG was much talked about on the Good Companions' email discussion list. It was agreed that the standard symbol for the AG in problem diagrams would be a Queen rotated 135 degrees clockwise. (If you're using a font that doesn't have this symbol, a Queen rotated 90 degrees clockwise is recommended. If you don't have this either, you can use an upside-down Queen, but this is to be avoided if possible since the upside-down Queen is already the standard symbol for the regular Grasshopper.)
Many people didn't like the dry and cumbersome name of Andernach-Grasshopper, and a lot of suggestions were offered for renaming the piece. Although the name is in fact dry and cumbersome, ultimately I had to reject all other proposals. The problem with all suggested naming systems was that the did not account for the number of possible pieces that can be created. The Andernach-Grasshopper concept can be extended to virtually any piece that is required to leap over another piece, including, but not limited to, the Bishop-Lion, Bishopper, Contragrasshopper, Double-Grasshopper, Eagle, Eagle-Lion, Equihopper, Kangaroo, Leo, Lion, Moose, Pao, Rookhopper, Rook-Lion, and Vao. The Einstein-Grasshopper and Circe-Grasshopper can be extended to all these pieces as well. Additionally there are combination pieces, such as the Andernach-Circe-Grasshopper and the Einstein-Andernach-Grasshopper (more on these below).
The Andernach-Grasshopper was added to the free problem-solving program Popeye in March of 2001 (the link may be down for the moment, as the organization hosting it is in the process of moving).
Movement
The Andernach-Grasshopper moves likes a Grasshopper, except that the piece it hurdles changes colors, except for Kings.More specifically, the AG moves any number of squares horizontally, vertically, or diagonally until it reaches an occupied square. It then jumps over this piece to the square immediately beyond it. The piece jumped over - if it is not a King - it changed to the opposite color of what it was when the AG began the move. The AG may only move to unoccupied squares or squares occupied by an enemy piece, which is then captured.
The AG was inspired by and takes its name from Andernach Chess, a fairy condition that has been popular with problemists for some time.
Movement Diagram
In the diagram below, the white AG on d4 can move to any of the squares marked with a green circle. If it moves to f6, the black AG on e5 becomes white. If it moves to a1, the white pawn on b2 becomes black. If the AG jumps either King, they stay the same color. The AG can also capture the Rook on d7, changing the Queen on d6 from black to white, which then gives check to the black King.The AG can not move to the square marked with a red circle, because in doing so it would change the Bishop on b6 to black, giving check to the white King. The AG can not jump the white Rook on g7 because it can only hurdle the first piece it reaches. It can not jump the white Pawn on g4 because the Pawn on h4 blocks it. The AG can not move straight downward because there is nothing in that direction for it to hurdle.

Remarks
I invented the Andernach-Grasshopper as a problem piece. As a game piece it is probably less useful, since grasshopper-type pieces are known as being less mobile since they depend on other pieces. More useful for playing purposes would be a piece that is more mobile, less awkward, and only changes the color of enemy pieces and not friendly pieces.Another idea I proposed is that of the 'rex inclusive' AG, in which Kings would also be affected by the Andernach-Grasshopper. In problems then, a player could only be checkmated if he actually had a king of his color on the board, and a player could have more than one king at a time. I think this idea has a lot of potential, but it did not receive much support on the Good Compantions discussion list.
Problems
Problem 1This problem is by Milan R. Vukevich. It received first place in the Good Companions 2000 quick-composing tourney. You can read the solution and comments here. The problem is selfmate in 2, meaning white is trying to force black to checkmate him in 2 moves.
Prize
Good Companions 2000 QCT
s#2
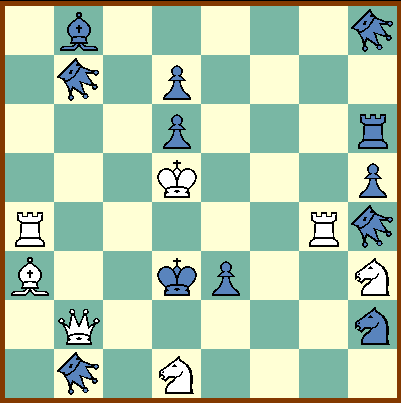
Problem 2
This problem is by David L. Brown. It received first place in the USA
vs GB Composing Match for AG Helpmates. You can read the solution and
comments here. The problem is a
helpmate in 3, meaning white and black must cooperate to achieve
checkmate in 3 moves, with black moving first.
Problem 3
This problem is by Dan Meinking. It appeared in the StrateGems issue
17. You can read the solution and comments here. The problem is a helpmate in
5-1/2, meaning white and black must cooperate to achieve checkmate in
5-1/2 moves, with white moving first.
If the EG moves without capturing, the piece it hurdles decreases
according to the following heirachy: EG-P-N-B-R-Q. Since there is
nothing below EG, if an EG hurdles another EG without capturing, the
hurdled piece is unaffected.
If the EG captures a piece on its move, the piece it hurdles increases
according to the following heirachy: P-N-B-R-Q-EG. Since there is
nothing above EG, if an EG hurdles another EG and captures, the
hurdled piece is unaffected.
Note that Kings are never affected by the EG.
This idea is based on Einstein
chess, a fairy condition known among problemists. Some people did not
like that I put the EG on either end of the list, but I did this
according to the already-existing standard for fairy pieces in
Einstein chess.
The EG on d4 can not move to the square marked with a red circle,
because in doing so it would change the Bishop on e5 to Knight, which
would then give check to the white King.
The black EG on h8 can move to f8, changing the Knight on g8 to Pawn.
A player who has a Pawn on his own first rank may move that Pawn 1, 2,
or 3 squares on its initial move.
The black EG on c1 can move to f1, changing the Knight on e1 to Pawn.
A Pawn created in this way does not automatically promote; instead, it
is immobile until it is changed to something else.
1st Place
USA vs GB Composing Match -
Section I
h#3 b)bBe3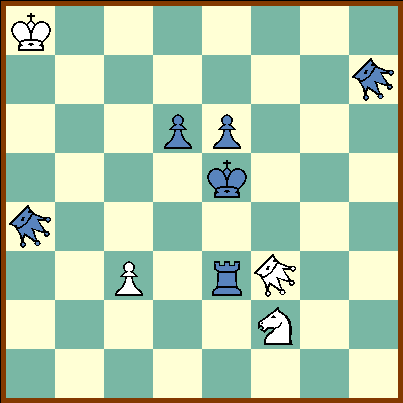
StrateGems 2002
h#5-1/2
The Einstein-Grasshopper
The EG moves the same as the Andernach-Grasshopper; that is, like a
grasshopper. Unlike the AG, however, the EG does not change the color
of the pieces it hurdles. Instead, it changes what piece they are,
according to the following rules:
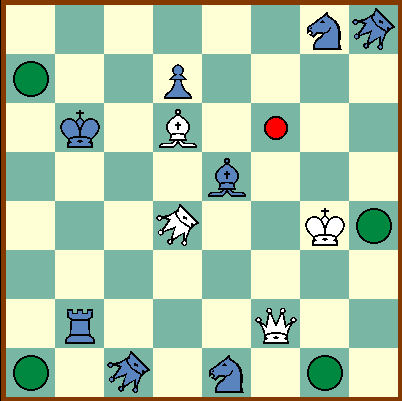
Movement Diagram
The Einstein-Grasshopper is represented in the diagram below by a
queen rotated 135 degrees counterclockwise. In the diagram, the white
EG on d4 can move to any of the squares marked by a green circle. If
it moves to a1, the Rook on b2 decreases to Bishop. If it moves to
g1, the Queen on f2 decreases to Rook. If it moves to h4 or a7, the
Kings are unchanged. The EG can also capture the black Pawn on d7,
changing the Bishop on d6 to Rook, which then gives check to the black
King.
Problem
The EG has not yet been used in any published problems. The problem
below is one I composed to demonstrate the EG. It has not been
computer-tested and whether or not the problem is sound is unknown.
The problem is a series-selfmate in 20, meaning white get 20 moves in
a row, and must set up a position so that black is forced to checkmate
him on the next move. You can see the solution here.
unpublished, 2000
ser.s#20, C-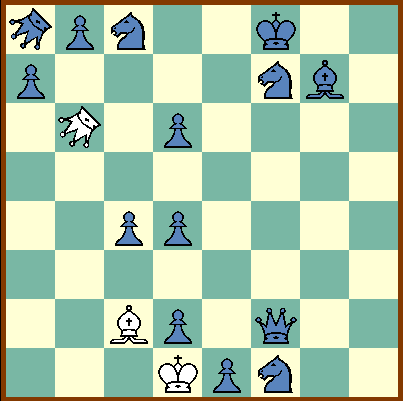
The Circe-Grasshopper
Like the AG and EG, the Circe-Grasshopper moves like a grasshopper. Pieces hurdled by the CG, however, are replaced on the board according to the rules of Circe Chess (also see Circe). More specifically, when a CG hurdles a piece, that piece is placed on its rebirth square according to the following rules:The rebirth square of a piece is the square that that piece would have occupied at the beginning of a standard chess game.
-
A Rook, Knight, or Bishop is reborn on the same color square as the
one on which it was hurdled.
-
A pawn is reborn on the same file as the one on which it was hurdled.
-
All fairy pieces are considered promotions, therefore they are
reborn on the last rank for the file on which they were hurdled.
-
A reborn King or Rook has the option of castling.
- If a piece's rebirth square is occupied, that piece is removed from the game. If a King's rebirth square is occupied, he is checkmated.
Movement Diagram
The Circe-Grasshopper is represented in the diagram below by a Queen rotated 45 degrees clockwise. In the diagram, the CG on b4 can move to any of the squares marked with a green circle. If the CG moves to g4, the black King on f4 would be reborn on e8. If the CG moves to d2, the black CG on c3 would be reborn on c1. The CG can move to f8, sending the white Bishop on e7 to c1, which then gives check to the black King. If the CG moves to b7, the white Pawn on b6 is removed from the game because the Pawn's rebirth square - b2 - is occupied by the black Rook.The CG can not move to the square marked with a red circle. Doing so would send the black Rook on b2 to h8, where it would give check to the white King.
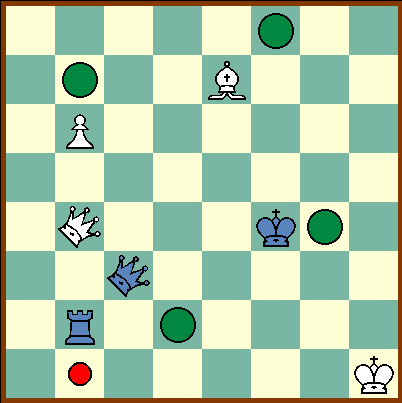
There are currently no published problems using the Circe-Grasshopper, and I don't have any good demonstration problems at this time.
Combination Pieces
Using the three pieces already described, it is possible to create several combination pieces. These include the following:
Andernach-Circe-Grasshopper
Andernach-Einstein-Grasshopper
Circe-Andernach-Grasshopper
Circe-Einstein-Grasshopper
Einstein-Circe-Grasshopper
Andernach-Circe-Einstein-Grasshopper
Andernach-Einstein-Circe-Grasshopper
Circe-Andernach-Einstein-Grasshopper
Einstein-Circe-Andernach-Grasshopper
In each case, the grasshopper has multiple effects on the piece that it hurdles, which are considered to occur in the order they are listed in the piece's name. For example, an ACG hurdling a white Queen would relocate it to d8, because it would be changed from white to black first, and then be reborn according to the rules of Circe. But a CAG hurdling the same white Queen would relocate it to d1 first, then change it to black. And that same white Queen hurdled by a noncapturing CEG would be reborn on d1 as well, but this time as a white Rook. Yet an ECG would send relocate the Queen to either a1 or h1, depending on the color square she was on at the time of the hurdle. Note that order is only a factor when Circe is involved, therefore the AEG and EAG are the same piece, as are the CEAG and the CAEG
Final Remarks
The page has largely focused on grasshopper forms of these pieces, because a) that's how I originally thought of them, b) the grasshopper is one of the most popular fairy chess pieces of all time, and definitely the most popular hurdler, and c) the Andernach-Grasshopper has been used in published problems and tournaments. As I mentioned in the historical notes, however, there is a virtually unlimited supply of pieces that can be adapted to these ideas. I look forward to seeing problems that use such pieces as the Andernach-Kangaroo, the Einstein-DoubleGrasshopper, and the Circe-Leo.Additionally, these pieces can be combined with regular fairy conditions - the most obvious combinations being AG's in an Andernach problem, EG's in an Einstein problem, and CG's in a Circe problem. And of course, any of the triple-combination pieces in a problem that is Andernach, Einstein, and Circe.
I have yet to invent or discover any other fairy condition that can be adapted to hurdlers in the same way as these three.
This is an item in the Piececlopedia: an overview of different (fairy) chess pieces.
Written by Benjamin C Good.
WWW page created: August 14th, 2002.